Horst Kunow, mailto:hkunow@kernphysik.uni-kiel.de
Bernd Heber, mailto:bheber@kernphysik.uni-kiel.de
Reinhold Müller-Mellin, mailto:mueller-mellin@kernphysik.uni-kiel.de
Kiel,
March 10, 1998
The Kiel Electron Telescope Sensor System
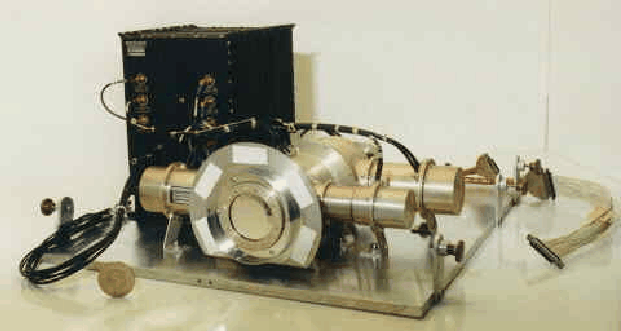
This figure displays in front the flight spare model sensor unit of the Ulysses Kiel Electron Telescope (KET) and in the back the electronics box. For comparison a five German mark coin is shown to demonstrate the size of the instrument. The KET is part of the Ulysses cosmic ray and solar particle investigation (COSPIN) experiment, which has been described in detail by Simpson et al. [4].
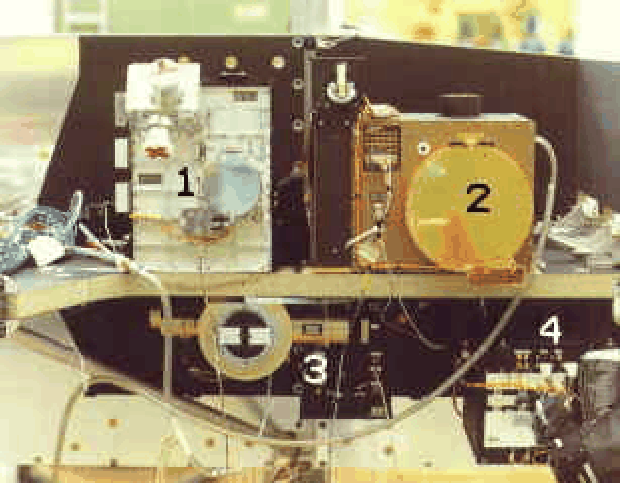
This figure shows the COSPIN sensor units as they are mounted on the spacecraft. The KET (3) is mounted below the Low Energy Telescope (LET), the Anisotropy Telescope (AT), the High Energy Telescope (HET) and the High Flux Telescope (HFT).

This figure shows a schematic sketch of the KET sensor system. D1 and D2 are 0.5 mm thick semiconductor detectors, C1 is an aerogel Cerenkov-detector and A a plastic anticoincidence scintillator. C2 is a lead fluoride Cerenkov-detector and S2 a plastic scintillator. PM1 through PM4 are photomultipliers.
Functionally, the detector system consists of two parts: an entrance telescope and a calorimeter, surrounded by a guard counter A.
The KET is designed to measure electron, proton and alpha particle fluxes in several energy windows ranging from a few MeV/n up to and above a few GeV/n. The values listed in the following table are based on mean energy losses and geometry of the instrument.
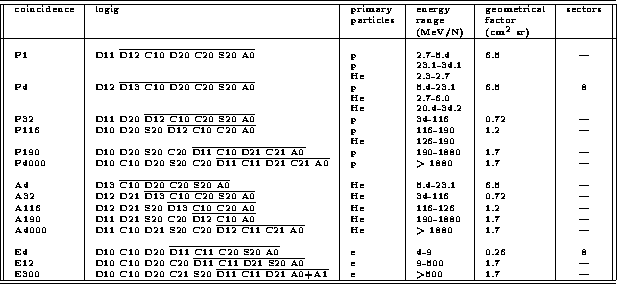
This table summarizes the KET coincidence channels. The coincidence name and trigger condition are displayed in the first two columns. The particles and their corresponding energy range are listed in columns three and four. The geometrical factor and sectorization information of the coincidence channels are in columns five and six.
Monte-Carlo-Simulation of the KET
A treatment of the response functions of particle telescopes, and a number of exact formulae for multi-element telescopes have been given by Sullivan, [5]. However, the determination of the response function of rather complex telescopes like the KET instrument, makes a Monte Carlo simulation mandatory. We assume that the differential coincidence counting rate of a particle telescope can be expressed as:
where dCi,k is the differential coincidence counting rate in channel i, Jk(E) the flux of particle species k with kinetic energy E, and Rik(E) the response function for particle species k in channel i, to be determined by the simulation. To get the counting rate Ci for the channel i we have to integrate over E and sum up for all particle species. In general, the response function may depend on many variables like the angular distribution of the incident particle flux, the location where a particle penetrates a detector etc. Here we assume that R(E) is a function only of kinetic energy, valid for particle fluxes which are almost isotropic over the effective opening angle, and that the simulation properly averages over all other dependencies.
The Monte Carlo simulation was performed with the CERN Library Program GEANT 3 (BRUN et al., [1]). Particles were followed down to a low energy cutoff (electrons and gamma-rays 50 keV, protons 300 keV), once reaching this cutoff the particles were considered to be stopped and to have deposited all of their kinetic energy in the traversed material. The geometry of the detectors, mountings, foils, and the relevant structure material as well as the energy resolution of the readout electronics were accounted for in the simulation.
The model of the KET sensor unit in the simulation. Shown are three possible tracks of 120 MeV protons. Two of these tracks are triggering the channel P32 and on of them is counted in P116.
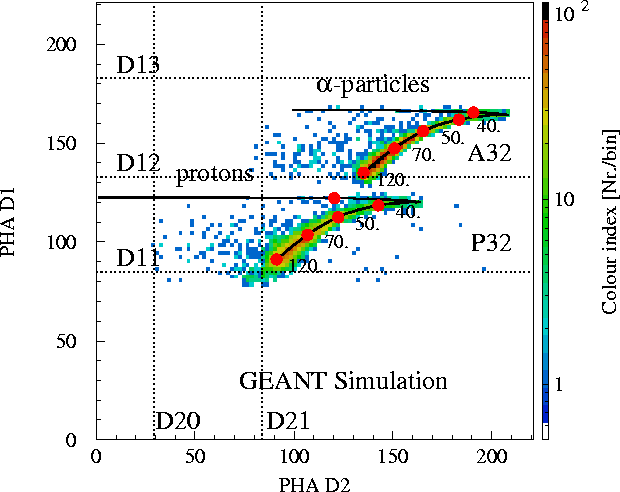
Calculated D1-D2 PHA-distribution (energy loss), using a proton and alpha distribution which is a function of energy and isotropic in direction. The solid lines show the mean energy losses. Marked on these lines are the expected energy losses for 35, 40, 50, 70 and 120 MeV/n. The dotted lines display the electronics thresholds. In comparison the following figure shows a PHA distribution measured in space:

Measured P32-protons and A32-alpha-particle D1-D2 matrix in January 1991. The entries below the dotted line could be identified as background random coincidences (HEBER, [2]). As is discussed in detail in HEBER, [2], the proton and alpha-tracks in that matrix are well described by the Monte-Carlo simulation. One important result of the analysis are the determination of the geometrical factors as a function of energy:
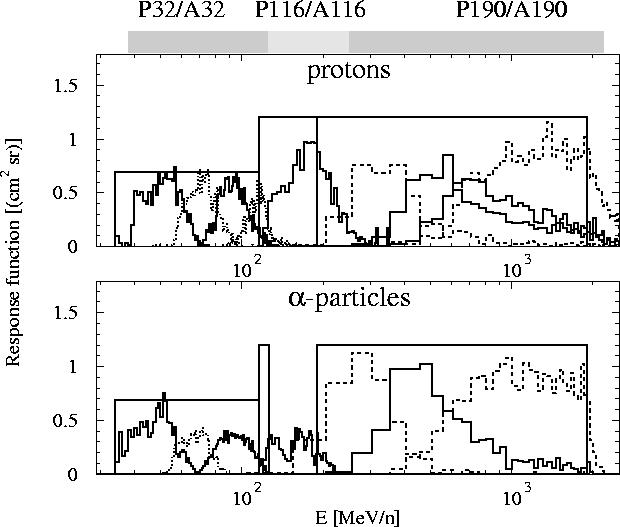
Response functions RSimi(E) for isotropic protons and alpha-particles. The rectangular boxes indicate the response functions expected by using the Sullivan [5] theory. For the low energy channels this theory is a good approximation.
| G/(cm2 sr MeV/n) | E /(MeV)/n | Sigma;E/(MeV)/n | |
| protons K3 | |||
| S | 57.8 | 81.0 | 26.1 |
| alpha-particles K33 | |||
| S | 52.7 | 78.2 | 24.0 |
| protons K34 | |||
| S | 83.3 | 190 | 42 |
| alpha-particles K29 | |||
| S | 23.4 | 164 | 25 |
| protons K12 | |||
| 1 | 230 | 315 | 160 |
| 2 | 500 | 700 | 390 |
| 3 | 1370 | 1250 | 420 |
| 4 | 1500 | 950 | 490 |
| alpha-particles K31 | |||
| A | 220 | 315 | 150 |
| B | 480 | 700 | 390 |
| C | 1320 | 1250 | 420 |
| D | 1500 | 950 | 490 |
KIEL ELECTRON TELESCOPE readme
All Ulysses data system files (UDS files) have the name
Herein YY is the year (eg. 90) and DOY the day of the year (eg. 365 of year 90 is 31.12.90).
The KET files are written on a VMS machine using Fortran 77 Routines. The format used is:
IMPLICIT REAL(K) WRITE(40,'(6I5)') IYEAR,IDOY,IHOUR,IMIN,ISEC,ICOV WRITE(40,'(10G11.3)') 1 K1,K21,K22,K23,K24,K25,K26,K27,K28,P4 WRITE(40,'(10G11.3)') 1 K3,K34,K12,K10,K2,K33,K29,K31,K30,K13 WRITE(40,'(10G11.3)') 1 K14,K15,K16,K17,K18,K19,K20,E4,K11,K32 WRITE(40,'(6G11.3)') 1 D10,D20,C10,C20,A01
Parameters are:
| IYEAR: | year | |
| IDOY: | day of year | |
| IHOUR: | hour | |
| IMIN: | minute | |
| ISEC: | second | |
| ICOV | coverage in percent | |
| KET channel | energy range A&A | energy range |
| Monte-Carlo simulation | ||
| K1: | protons (2.7-5.4 MeV) | |
| K21-K28: | " (5.4-23.1 MeV) sectors 1 through 8 | |
| P4: | " (5.4-23.1 MeV) omnidirectional | |
| K3: | " (34.1-125.0 MeV) | |
| K34: | " (125.0-320.0 MeV) | (125.0-250.0 MeV) |
| backward penetrating particles (160.0-260.0 MeV) | ||
| K12: | " (320.0-2100.0 MeV | (250.0-2200.0 MeV) |
| backward penetrating particles (260.0-2200.0 MeV) | ||
| K10: | " (>2100.0 MeV) | (>2200.0 MeV) |
| backward penetrating particles (>2200.0 MEV) | ||
| K2: | helium (6.0-20.4 MeV) | |
| K33: | " (34.2-125.0 MeV) | |
| K29: | " (125.0-320.0 MeV) | (125.0-155.0 MeV) |
| backward penetrating particles (155.0-225.0 MeV) | ||
| K31: | " (320.0-2100.0 MeV) | (250.0-2100.0 MeV) |
| backward penetrating particles (250.0-2100.0 MeV) | ||
| K30: | " (>2100.0 MeV) | |
| K13-K20: | electrons (2.5-7.0 MeV) sectors 1 through 8 | |
| E4: | " (2.5-7.0 MeV) omnidirectional | |
| K11: | " (7.0-170.0 MeV) | |
| K32: | " (>170.0 MeV) | |
| D10 - A01: | single detector count rates |
| KET channel | Gi |
| K1 | 18 |
| K21 - K28 | 120 |
| P4 | 120 |
| K3 | 70.0 |
| K34 | 152.0 |
| K12 | 3300.0 |
| K10 | na |
| K2 | 120 |
| K33 | 70.0 |
| K29 | 88.0 |
| K31 | 3200.0 |
| K13-K20 | na |
| E4 | na |
| K11 | na |
| K32 | na |
===============================================================
GET LOW FLUX VALUES FROM THE PI ON LONGER ACCUMULATION PERIODS
===============================================================
The daily averaged and 27 day averaged data are also provided. See readme.daily 3.3 and readme.27days 3.4.
===============================================================
Levels below 3.0e-3, 2.5e-3 should not be considered.
===============================================================
=================================================
Caution: Very high fluxes for KET !!!!
=================================================
The daily averaged file KET90-96.DAILY contains a subset of KET coincidence channels which can be corrected by using Pulse Height Analysis (see Heber [2]). As an example the masks choosen for K3 and K34 are shown in the following figure.

Definition of PHA masks in K3, K33 Matrix using the result of a GEANT simulation.
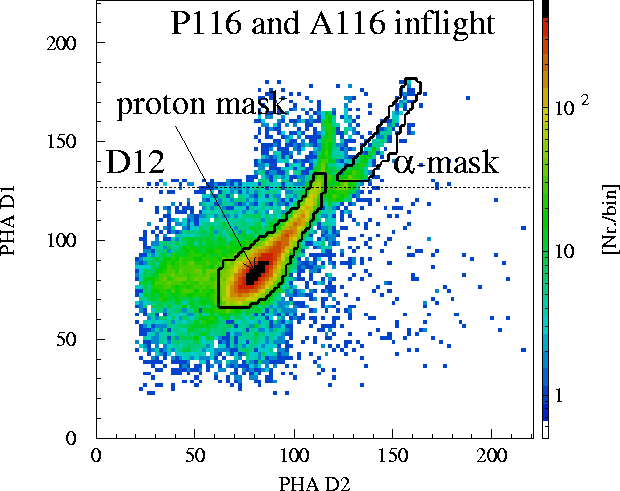
Definition of PHA masks in K34, K29 Matrix using the result of a GEANT simulation. In contrast to the upper panel inflight data are shown. Note that we used a for this figure a time period of 30 days. Because of the low PHA-statistics for K29 no daily averaged corrected rates are given on a one day basis.
The KET file was written on a VMS machine using Fortran 77 Routines. The format used is:
IMPLICIT REAL(K)
WRITE(40,'(I2,1X,I3,1X,I3,1X,8G10.3/10X,6G10.3)')
1 IYEAR,IDOY,ICOV
1 K3,EK3,K34,EK34,K12,EK12,K10,EK10,
1 K33,EK33,K31,EK34,K30,EK30
Parameters are:
| IYEAR: | year | |
| IDOY: | day of year | |
| ICOV | coverage in percent | |
| KET channel | energy range A&A | energy range |
| Monte-Carlo simulation | ||
| K3: | protons (34.1-125.0 MeV) | |
| EK3: | error of preceeding value | |
| K34: | " (125.0-320.0 MeV) | (125.0-250.0 MeV) |
| backward penetrating particles (160.0-260.0 MeV) | ||
| EK34: | error of preceeding value | |
| K12: | " (320.0-2100.0 MeV | (250.0-2200.0 MeV) |
| backward penetrating particles (260.0-2200.0 MeV) | ||
| EK12: | error of preceeding value | |
| K10: | " (>2100.0 MeV) | (>2200.0 MeV) |
| backward penetrating particles (>2200.0 MEV) | ||
| EK10: | error of preceeding value | |
| K33: | helium " (34.2-125.0 MeV) | |
| EK33: | error of preceeding value | |
| K31: | " (320.0-2100.0 MeV) | (250.0-2100.0 MeV) |
| backward penetrating particles (250.0-2100.0 MeV) | ||
| EK31: | error of preceeding value | |
| K30: | " (>2100.0 MeV) | |
| EK30: | error of preceeding value |
The KET files are written on a VMS machine using Fortran 77 Routines. The format used is:
IMPLICIT REAL(K)
WRITE(40,'(4I3,2G13.4)') IYEAR,IDOY,IHOUR,IMIN,E4,EE4
Parameters are:
| IYEAR: | year | |
| IDOY: | day of year | |
| IHOUR: | hour | |
| IMIN: | minute | |
| KET channel | energy range A&A | energy range |
| Monte-Carlo simulation | ||
| E4: | " (2.5-7.0 MeV) omnidirectional | |
| EE4: | error of preceeding value |
The translation was initiated by Bernd Heber on Tue Mar 10 17:17:11 MET 1998